1) two-dimensional parabolic equation


二维抛物型方程
1.
The initial-value problem of two-dimensional parabolic equation can be solved by using meticulous integration of single inner point subdomain.
可用单内点子域精细积分法 ,求解二维抛物型方程初值问题 。
2.
A class of two-level explicit difference schemes are presented for solving two-dimensional parabolic equation.
构造了一族二维抛物型方程的一族两层显式格式 ,当截断误差为O(Δt+Δx2 )时 ,稳定性条件为网比r =Δt/Δx2 =Δt/Δy2 ≤ 1/ 2 ,优于同类的其他显式格式 ,当截断误差为O(Δt2 +Δx4 )时成为一个简洁而实用的高精度两层显式格式 。
2) two-dimensional parabolic partial differential equation


二维抛物型偏微分方程
3) one-dimensional parabolic equation


一维抛物型方程
1.
A high accurate semi-explicit difference method for the one-dimensional parabolic equation;
求解一维抛物型方程的一种高精度半显式差分方法
2.
One implicit differencing scheme of three level and seven points for solving the one-dimensional parabolic equations is presented in this paper,by using the method of combinatorial difference quotient.
用组合差商法对一维抛物型方程构造了一个两层七点差分隐格式,使得精度达到O(2τ+h4),稳定性条件为0≤r≤1/3。
4) one-dimension parabolic equation


一维抛物型方程
1.
One class of high accuracy explicit difference schemes of three layers and seven points for solving one-dimension parabolic equations are presented by the undetermined parameters method,its truncation error is O(τ3+h6),the stability condition isO<r4/5.
利用待定系数法对一维抛物型方程构造了一类高精度的三层七点显式差分格式,格式的截断误差达到O(τ3+h6),稳定性条件是0
2.
One implicit difference scheme for solving one-dimension parabolic equations is presented in this paper.
本文用组合差商法在乘积型差商空间中对一维抛物型方程初边值问题构造了一个绝对稳定的隐式差分格式,格式的截断误差阶为O(τ2+h4)。
5) three-dimension parabolic equation


3维抛物型方程
6) parabolic equation in multi-dimension


高维抛物型方程
1.
By using the addition of dissipation term, the author establishes some three-layer explicit difference schemes for solving parabolic equation in multi-dimension, these schemes are absolutely stable , which extend the results of paper [1] .
本文利用加耗散项的方法,建立了高维抛物型方程的若干恒稳的三层显式差分格式,推广了文[1]的结果。
补充资料:抛物型偏微分方程
| 抛物型偏微分方程 parabolic type,partial differential equation of 偏微分方程的一类。最典型的是热传导方程 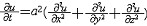 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式 (a>0) (1)基本解是点热源的影响函数。若在t=0时在(ξ,η,ζ)处给定单位点热源,即u0(x0,y0,z0,0)=δ(ξ,η,ζ)(δ为狄拉克函数),则当t>0时便引起在R3的温度分布,这就是基本解。用傅里叶变换可得到它的表达式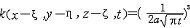 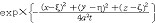 热传导方程初值问题的解可用基本解叠加而成,即 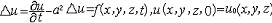 的解为 的解为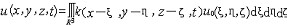  极值原理:一个内部有热源的传导过程,它的最低温度一定在边界上或初始时刻达到。更强的结论是 :如果t=T时在Ω内某一点达到最低温度 ,则在这个时刻以前(t<T时)u≡常数 ;又:若最低温度在t=T时边界¶Ω上某点P达到,则在这点上 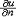 |P,Τ<0(n为外法线方向)。 |P,Τ<0(n为外法线方向)。 |
说明:补充资料仅用于学习参考,请勿用于其它任何用途。
参考词条